Conservation of mechanical energy on curve surface – problems and solutions

Conservation of mechanical energy on curve surface – problems and solutions

1. If ball’s speed at point A is 6 m/s, ball’s speed at point B is √92 m/s, and acceleration due to gravity is g = 10 m/s². What is the height of point B (h)?

Known :

Speed of ball at point A (v_A) = 6 m/s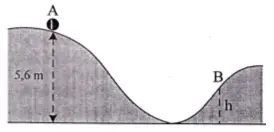

Speed of ball at point B (v_B) = √92 m/s

Acceleration due to gravity (g) = 10 m/s²

The height of A (h_A) = 5.6 meters

The height of B (h_B) = h

Wanted : The height of point B (h)

Solution :

The initial mechanical energy = the gravitational potential energy

At point A, ball’s speed = 0, so the kinetic energy of the ball = 0. KE = 1/2 m v² = 1/2 m (0) = 0.

Ball at the height of 5.6 meters so the ball has the gravitational potential energy. The gravitational potential energy : PE = m g h = m (10)(5.6) = 56 m

The initial mechanical energy = the gravitational potential energy + kinetic energy = 56 m + 0 = 56 m

The final mechanical energy = the gravitational potential energy + kinetic energy

At point B, the height of ball is h. The gravitational potential energy : PE = m g h = m (10) h = 10 m h

The kinetic energy of the ball : KE = 1/2 m v² = 1/2 m (√92)² = 1/2 m (92) = 46 m

The final mechanical energy = the gravitational potential energy + the kinetic energy = 10 m h + 46 m = m (10 h + 46)

The principle of conservation of mechanical energy :

The initial mechanical energy = the final mechanical energy

56 m = m (10 h + 46)

56 = 10 h + 46

56 – 46 = 10 h

10 = 10 h

h = 10/10

h = 1 meter

2.

If the initial velocity = 0, the acceleration due to gravity = 10 m/s², then what is the speed at the height of B.

Known :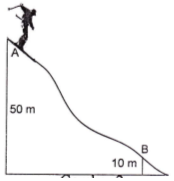

The initial speed (v_o) = 0

The initial height (h_o) = 50 m – 10 m = 40 m

The final height (h_t) = 0

Acceleration due to gravity (g) = 10 m.s^-2

Wanted : The final speed (v_t)

Solution :

The change of the kinetic energy :

ΔKE = 1/2 m (v_t² – v_o²) = 1/2 m (v_t² – 0) = 1/2 m v_t²

The change of the potential energy :

ΔPE = m g (h_t – h_o) = m (10)(0-40) = m (10)(-40) = – 400 m

Calculate the final speed (v_t) using the equation of the principle of conservation of mechanical energy :

0 = ΔKE + ΔPE

0 = 1/2 m v_t² – 400 m

1/2 m v_t² = 400 m

1/2 v_t² = 400

v_t² = 2(400)

v_t = √(400)(2)

v_t = 20√2 m/s

1. What is meant by “conservation of mechanical energy”?
   • Answer: The conservation of mechanical energy means that the total mechanical energy (sum of kinetic and potential energy) of an isolated system remains constant if only conservative forces, like gravity, act on it.
2. How does an object moving on a curved surface (like a ball rolling down a curved ramp) conserve its mechanical energy?
   • Answer: As the object moves down the curved surface, potential energy is converted into kinetic energy, but the total mechanical energy remains constant, assuming no non-conservative forces (like friction) are doing work.
3. If a ball is released from rest at the top of a curved ramp, how does its kinetic energy change as it moves down the ramp?
   • Answer: The ball’s kinetic energy increases as it moves down the ramp because its potential energy decreases and is converted into kinetic energy.
4. In the absence of friction, how would the speed of a ball at the bottom of a curved slide compare to a straight slide of the same height?
   • Answer: The speed of the ball at the bottom would be the same for both the curved and straight slides because the conversion of potential energy to kinetic energy depends only on the vertical height, not the path taken.
5. Why might a ball not reach its original height when rolled up a curved ramp after descending from a certain height?
   • Answer: If there are non-conservative forces like friction acting on the ball, they will do work against the motion, converting some of the ball’s mechanical energy into other forms, like heat. As a result, the ball might not have enough energy to reach its original height.
6. If two different shaped objects (e.g., a sphere and a cube) slide down a curved ramp from the same height without rolling, will they have the same speed at the bottom? Why?
   • Answer: Yes, they will have the same speed at the bottom. Assuming no rolling and no friction, the only factor determining their speed is the conservation of energy based on the initial height, not their shape.
7. How does the curvature of a surface affect the normal force on an object moving along it?
   • Answer: The curvature causes the direction of the normal force to change as the object moves. On a concave upward surface (like the inside of a bowl), the normal force is greater at the bottom due to the centripetal force required for curved motion. Conversely, on a convex surface, the normal force can decrease or even become zero (object leaves the surface) at the topmost point.
8. What role does the radius of curvature play in the conservation of mechanical energy on a curved surface?
   • Answer: The radius of curvature itself doesn’t directly affect the conservation of mechanical energy. However, it can influence the normal force and, if there’s friction, the amount of energy lost to non-conservative forces.
9. If a track has a dip in the middle (like a U-shaped curve), how does the mechanical energy of an object vary as it moves through the dip and up the other side?
   • Answer: The object’s potential energy will decrease as it moves into the dip and increase its kinetic energy. As it moves up the other side, its kinetic energy will decrease, and its potential energy will increase. If only conservative forces act, the total mechanical energy remains constant throughout the motion.
10. Can an object have both translational and rotational kinetic energy when moving on a curved surface?

• Answer: Yes, if an object like a ball rolls (without slipping) on a curved surface, it will have translational kinetic energy due to its center of mass moving and rotational kinetic energy due to its rotation about its axis.