Article about Pressure in fluids
In physics, the pressure is defined as force per unit area, where the direction of the force is perpendicular to the surface area. Mathematically, pressure is expressed by the equation P = F / A, where P = pressure, F = force and A = surface area. Force unit (F) is Newton (N), the unit of area is the square meter (m2). Because pressure is the force per unit area, the pressure unit is N/m2. Another name for N/m2 is Pascal (Pa). Pascal was used as a pressure unit to honor Blaise Pascal.
When the fluid is at rest, the fluid provides a force that is perpendicular to the entire surface of the contact. For example, we review the water in the glass; each part of the water provides a force with a direction perpendicular to the glass wall. So each section of the water provides a force perpendicular to each unit area of the container it occupies. This is one of the essential properties of the static fluid. When the fluid exerts an action force on the surface, where the direction of the force is not perpendicular, the surface will give a reaction force whose direction is not perpendicular. This will cause the fluid to flow. But the flu, id is at rest. So the conclusion is, in a stationary fluid, the direction of the force is always perpendicular to the surface of the container.
Another important property of the static fluid is that the fluid always exerts pressure in all directions. To better understand this explanation, insert an object that can float into the water in a container. If the water is at rest, then the object is not moving because the same pressure works on the entire surface of the object. If the water pressure is not the same, then there will be a total force, which will cause the object to move.
Effect of depth on pressure
How does the depth or height affect fluid pressure? Is seawater pressure at a depth of 10 meters the same as seawater pressure at a depth of 100 meters? Let’s review the water pressure in a container as shown in the figure. The height of the liquid column is h and the cross-sectional area A. What is the water pressure at the bottom of the container?
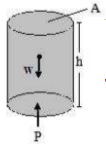w = weight of water, h = height of the water column in a cylindrical container, A = surface area, and P is pressure.
The mass of the liquid column is:
ρ = m / V
m = ρ V
m = ρ h A
The weight of liquid column is:
w = m g = ρ h A g
w = weight, ρ = density, m = mass, g = acceleration of gravity, V = h A = volume of liquid (h = height, A = surface area)
If the equation above inserted into the equation of pressure :
p = ρ g h → Equation 1 (closed container)
p = pa + ρ g h → Equation 2 (open container)
pa = atmospheric pressure, ρ g h = hydrostatic pressure
If the open container contains water, atmospheric pressure is directed downward on the surface of the water.
Based on the equation above, the pressure is directly proportional to the density and depth of the liquid (gravity acceleration is constant).
If the height difference is huge (for example in very deep seawater), the density is slightly different. But if the difference in height is not too significant, basically the density of the liquid is the same, or the difference is so small that it is ignored.
We can also use the above equation to calculate the pressure difference at each different depth. The above equation is written again:
Δp = ρ g Δh
Δp = difference in pressure, Δh = difference in height
Atmospheric Pressure
When we dive into the water, all parts of our body are covered with water. The deeper we dive, the greater the pressure we feel. Every day we are also covered by the atmosphere which always suppresses all parts of our body like when we are in the water. As in seawater, the surface of the earth can be compared to the “sea-based” of the atmosphere. If the atmosphere suppresses all parts of our body at any time, why don’t we feel it, as if we were at the base of the sea? Our body cells maintain inside pressure that is almost the same as external pressure. This makes us not feel the effects of these pressure differences.
Earth’s atmospheric pressure also changes with height. But the earth’s atmospheric pressure is different from the liquid. Changes in density of liquids are very small for differences in depth that are not very large so that the density of the liquid is considered the same. This is different from the mass of the earth’s atmosphere. The mass of the earth’s atmosphere varies considerably with altitude. The density of air at each altitude varies so that we cannot calculate atmospheric pressure using the equations that have been derived above. Also, there is no limit on atmospheric from which the heights can be measured. Atmospheric pressure also varies with the weather. To determine atmospheric pressure, we take measurements.
Measurement of Pressure
Evangelista Torricelli (1608-1647), a student of Galileo, made a way to measure atmospheric pressure in 1643 using the mercury barometer of his work. The barometer is a long glass tube, in which the tube is filled with mercury. Well, the glass tube containing mercury is reversed in a dish that has also been filled with mercury.
When a glass pipe containing mercury is reversed, the end of the tube is not filled with mercury; the contents are only mercury vapor, the pressure is so small that it is ignored (p2 = 0). On the surface of mercury that is inside the plate, there is an atmospheric pressure which is directed downward (the atmosphere presses the mercury on the plate). The atmospheric pressure holds the mercury column in the glass pipe. In the figure, atmospheric pressure is represented by po. The amount of atmospheric pressure can be calculated using the equation:
po = ρ g h
Based on the result of measurement, the average of the atmospheric pressure at sea level is 1.013 x 105 N/m2. The amount of atmospheric pressure at sea level is used to define other pressure units, namely atm (atmosphere). So 1 atm = 1.013 x 105 N/m2 = 101.3 kPa (kPa = kilo pascal). Another pressure unit is a bar (often used in meteorology). 1 bar = 1.00 x 105 N / m2 = 100 kPa.
How is the atmospheric pressure above obtained?
The measurement uses the principles shown by Torricelli above. The mercury column height used is 76 cm (atmospheric pressure can only holds the mercury column which reaches only 76.0 cm in height), where the mercury temperature is used exactly 0 oC and the magnitude of the acceleration of gravity is 9.8 m/s2. The mass of mercury in this condition is 13.6 x 103 kg/m3. Atmospheric pressure:
po = ρ g h
po = (13.6 x 103 kg/m3)(9.8 m/s2)(76 cm)
po = (13.6 x 103 kg/m3)(9.8 m/s2)(76 x 10-2 m)
po = 101.3 x 103 N/m2
po = 1.013 x 105 N/m2
po = 1 atm
Pressure tools
There are many tools used to measure pressure, including open tube manometers.
In an open tube manometer, where the tube is U-shaped, some of the tubes are filled with liquid (mercury or water). The measured pressure is associated with the difference in the two heights of the liquid that is filled into the tube. The amount of pressure is calculated using the equation:
p = pa + ρ g h
pa = atmospheric pressure
ρ g h = measured pressure
ρ = density of liquid
Generally not the product ρ g h calculated but the height of the liquid (h) because the pressure is sometimes given in millimeters of mercury (mm Hg) or millimeter of water (mm H2O). Another name mm hg is torr (in honor to Evangelista Torricelli).
In addition to manometers, there are also other gauges namely aneroid barometers, both mechanical and electrical, including tire pressure gauges, etc. The tool used by Torricelli to measure atmospheric pressure is also called a mercury barometer, in which a glass tube filled with mercury.
Gauge Pressure and Absolute Pressure
Absolute pressure = atmospheric pressure + measured pressure. So to get absolute pressure, we adding gauge pressure with atmospheric pressure. In other words, absolute pressure = total pressure. Mathematically can be written:
p = pa + pg
For example if the tire pressure we measure = 100 kPa, then the absolute pressure is:
p = pa + pg
p = 101 kPa + 100 kPa
p = 201 kPa
The amount of absolute pressure = 201 kPa
Example problem 1 :
A submarine dives to a depth of 200 meters. What is the pressure experienced by the submarine? g = 10 m/s2
Known :
Depth (h) = 200 m
Density of seawater (ρ) = 1.03 x 103 kg/m3 = 1030 kg/m3
Atmosphere pressure (pa) = 1 atm = 1 x 105 N/m2 = 1 x 105 (Kg m/s2)/m2 = 1 x 105 Kg/ms2
Acceleration of gravity (g) = 10 m/s2
Wanted :
Solution :
p = pa + ρ g h
p = 1 x 105 kg/ms2 + (1030 kg/m3)(10 m/s2)(200 m)
p = 1 x 105 kg/ms2 + 2060000 kg/ms2
p = 1 x 105 kg/ms2 + 20.6 x 105 kg/ms2
p = 21.6 x 105 kg/ms2
p = 21.6 x 105 N/m2
p = 21.6 x 105 Pa
Containeir is open so that atmospheric pressure is not included in the calculation.
Example problem 2 :
A water reservoir A full with a height of 10 meter filled with water. If the surface of the water reservoir closed, what is the water pressure at the bottom of the container? g = 10 m/s2
Known :
Height (h) = 10 m
Density of water (ρ) = 1,00 x 103 kg/m3 = 1000 kg/m3
Atmosphere pressure (pa) = 1 atm = 1 x 105 N/m2 = 1 x 105 (kg m/s2) / m2 = 1 x 105 Kg/ms2
Acceleration of gravity (g) = 10 m/s2
Wanted : Pressure
Solution :
p = ρ g h
p = (1000 kg/m3)(10 m/s2)(200 m)
p = 2000,000 kg/ms2
p = 20 x 105 kg/ms2
p = 20 x 105 N/m2
p = 20 x 105 Pa
The container is closed so that atmospheric pressure is not included in the calculation.
Example problem 3 :
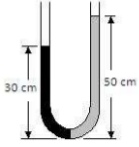The height of the water column (right) = 50 cm, while the height of the other liquid column (left) = 30 cm. What is the density of another liquid?
Known :
Acceleration of gravity (g) = 10 m/s2
Height of water (h1) = 50 cm
Density of water (ρ1) = 1 x 103 kg/m3 = 1000 kg/m3
The height of another liquid (h2) = 30 cm
Wanted : Density of another liquid
Solution :
pa + ρ1 g h1 = pa + ρ2 g h2
ρ1 h1 = ρ2 h2
ρ2 = ρ1 h1 / h2
ρ2 = (50 cm)(1000 kg/m3) / 30 cm
ρ2 = 1666.7 kg/m3
You must be logged in to post a comment.